Оригами ваза из модулей схема сборки пошагово: Модульное оригами ваза. Схема сборки вазы оригами
Модульное оригами ваза. Схема сборки вазы оригами
Схема сборки вазы в технике модульное оригами.
Здравствуйте уважаемые мастера и мастерицы! Представляю Вашему вниманию не сложную схему по сборке вазы в технике модульное оригами.


Для вазы потребуется 672 модуля размером 1/16. Из них:
Желтые — 287, Синие — 203, Красные — 91, Белые — 91.
Если Вы начинающий мастер модульного оригами, то рекомендую посмотреть эти статьи: «Как нарезать бумагу для модулей» и «Как сделать 3D модуль».
И еще, чтобы Вам уж совсем было легко собрать вазу, я записал небольшой видеоурок. Посмотрите, очень пригодится!




Смотрите внимательно фотографии и правильно считайте модули — НАЧНЕМ!


Первые три ряда из желтых модулей. В ряду 28 модулей.


4 ряд — увеличиваем количество модулей на 7. Каждый четвертый модуль увеличиваем. В ряду 35 модулей.


4 ряд — нужно заменить желтые на синие модули. Которые находятся по середине, т.е. между модулями которые увеличивали. В ряду 35 модулей.


5 ряд — модули, которые увеличивали, соединяем красными модули, остальное, как на фото. В ряду 35 модулей.


6 ряд — делаем синие ромбы.


7 ряд — в синем ромбике делаем красный. В ряду 35 модулей.


8 ряд — вставляем белую вставку. В ряду 35 модулей.


9 ряд — синие ромбики соединяются. В ряду 35 модулей.


10 ряд — ромбики начинаются сужаться.


11 ряд — красные модули соединились. В ряду 35 модулей.


12 ряд — красный ромб завершился.
13 ряд — завершаем синий ромб.


14 ряд — завершаем синие ромбики.
15 ряд — уменьшаем количество модулей на 7. Между ромбиками, производим уменьшение. Уменьшаем между всеми ромбиками. В ряду 28 модулей. Чтобы было понятнее — посмотрите видеоурок по изготовлению этой вазы.


16 ряд — уменьшаем количество модулей на 7. Уменьшение производится ровно над синими модулями каждого ромбика. Остальное заполняем синими модулями. В ряду 21 модуль.


17 ряд — вставляем синий модуль со смещением в лево от синего (модули вставляем обратной стороной). Далее желтый и белый, повторяем это до самого конца.


(1 фото) 19, 20, 21 ряды — продолжаем вставлять модули со смещением.
(2 фото) увеличиваем количество модулей в 2 раза. Вставляем по два модуля каждого цвета (синий, желтый, белый). В ряду 42 модуля.


Нужно раздвинуть модули последнего ряда в стороны, как на фото.


На этом заканчиваем собирать вазу в технике модульное оригами.


Ставьте лайки! Пишите комментарии! Делитесь в социальных сетях!
Модульное оригами схема вазы
Схема вазы, которую я Вам представляю, совсем другого формата, она намного сложнее предыдущих схем. Данная схема вазы, поможет Вам перейти на новый уровень. Модульное оригами — это бесконечное пространство Ваших фантазий, пользуйтесь этими возможностями, а я Вам в этом помогу. Я вас буду подталкивать, чтобы Вы могли быстрее подниматься по лестнице под названием «Мастер модульного оригами».


Для данной схемы вазы нам потребуется всего два цвета — оранжевый и фиолетовый. В самом начале, когда я выбрал данные цвета, я думал, что эти цвета не могут сочетаться, что будет не красиво (просто на это время у меня других цветов не было). Оказалось даже наоборот, получилось намного лучше, чем я думал. Модули для схемы вазы размером 1/16. И еще дно для вазы я делал из белых модулей.
Давайте начнем…


Далее будем вести отсчет рядов без дна, т.е. 1 ряд из фиолетовых модулей.


1-2 ряд — фиолетовый модули, в ряду 28 модулей.


3 ряд — каждый 4 модуль удваиваем, в ряду получается 35 фиолетовых модулей.


4 ряд — сейчас мы начинаем формировать узор.
В том месте, где удваивали модули, нужно вставить оранжевые модули, остальное заполняем фиолетовыми модулями. В ряду 35 модулей.


5 ряд — расширяем оранжевые модули, вставляем уже по 2 модуля. Между оранжевыми модулями, ровно по середине, снова увеличиваем ряд на 7 модулей, удваиваем каждый 5 модуль. Смотрите внимательно фотографию. В ряду 42 модуля.


6 ряд — там где удваивали модули, вставляем оранжевый модуль. Смотрите внимательно фото и повторяйте. В ряду 42 модуля, далее количество модулей в ряду меняться не будет.


7 ряд — у нас расширяется узор из оранжевых модулей.
8 ряд — должен получиться оранжевый ромб.


9 ряд — ромбы соединились между собой.
10 ряд — внутри большого ромба делаем маленький.
Далее будем ромб уменьшать.


11 ряд — смотрите внимательно фотографии.
12 ряд — маленький ромб завершаем и продолжаем уменьшать большой ромб.


13 ряд — продолжаем уменьшать ромб.
14 ряд — вставляем еще вставку из оранжевых модулей. Сейчас вазе можно придать форму бочонка.


15 ряд — ромбики соединяются (смотрите фото).
16 ряд — завершаем делать ромб и продолжаем делать оранжевые зигзаги.


17 ряд — продолжаем формировать зигзаги.


18 ряд — уменьшаем количество модулей в ряду по всему периметру. В ряду 28 модулей. Смотрите внимательно фото, чтобы все цвета совпадали.


19 ряд — завершаем делать зигзаг.
20 ряд — только фиолетовые модули. В ряду 28 модулей.


21 ряд — уменьшаем количество модулей в ряду на 7 модулей. Уменьшаем через 1 модуль, где начинать смотрите фото. В ряду 21 модуль.


22 ряд — только оранжевые модули.
23 ряд — фиолетовые модули вставленные обратной стороной.


24 ряд — в любом месте вставляем фиолетовый модуль, потом 2 оранжевых и так до самого конца.
25 ряд — один оранжевый и 2 фиолетовых модуля.


26 ряд — смотрите фото.
27 ряд — продолжаем формировать горловину.


28 ряд — получается небольшой зигзаг.
29 ряд — завершаем делать оранжевый зигзаг.


30 ряд — увеличиваем количество модулей в ряду через один модуль. В ряду 32 модуля.


31 ряд — вставляем фиолетовые модули, только наоборот. В ряду 32 модуля.


На этом завершаем делать вазу. Еще можно взять за горловину и немного придавить к низу, чтобы ваза немного расширилась.
Если у Вас возникли вопросы или имеются пожелания, то пишите в комментариях, я с удовольствием на них отвечу и Вам помогу. С уважением Сергей.
Модульное оригами схема вазы.
Сборка вазы оригами
Продолжаю серию по изготовлению великолепных двухцветных ваз оригами. Первый вариант двухцветной вазы оказался самым популярным среди всех схем этого блога. В связи с этим я решил продолжить сборку ваз оригами, а именно двухцветных.


Для того, чтобы собрать данную вазу, совсем не обязательно делать модули такого же цвета Можно применить совсем другие цвета, по главное, чтобы они были одного тона, например: темно-синий и голубой, темно-зеленый и салатовый.
Для этого потребуются модули размером 1/16, коричневые и желтые.


1-3 ряд — делаем дно из желтых модулей и два ряда коричневых. 28 модулей в ряду. Далее отсчет рядов будет вестись с коричневых модулей.


3 ряд — только желтые модули. Прибавляем количество модулей через каждые 3 модуля. В ряду 35 модулей.


4 ряд — вставляем только коричневые модули. В ряду 35 модулей.


5 ряд — Каждый 5 модуль желтый. В ряду 35 модулей.


6 ряд — желтых модулей вставляем по два. Еще нужно увеличить количество модулей в ряду на 7 модулей. Увеличиваем, как раз между желтыми модулями — по середине. В ряду 42 модуля.


7 ряд — желтыми модулями соединяем модули которые увеличивали. Смотрите внимательно фото.


8 ряд — ромб начинаем сужать. В ряду 42 модуля.


9 ряд — завершаем делать ромбики и продолжаем делать зигзаги.


10 ряд — Продолжаем делать зигзаги.


11 ряд — по середине вставляем желтые модули, начинаем делать новые зигзаги.


12 ряд — смотрите внимательно фотографии.


13 ряд — первый зигзаг завершился, продолжаем делать второй.


14 ряд — продолжаем делать второй зигзаг.


15 ряд — между коричневые вставляем желтые модули — это будут новые ромбики.


16 ряд — продолжаем формировать ромбики.


17 ряд — второй зигзаг завершился. Продолжаем делать ромб.


18 ряд — ромб начинаем сужать.


19 ряд — завершаем делать ромб.


20 ряд — только коричневые модули.


21 ряд — уменьшаем количество модулей по всему периметру. В ряду 28 модулей.


22 ряд — только коричневые модули.
23 ряд — вставляем модули через один.


24 ряд — уменьшаем количество модулей по всему периметру без пробелов. В конце остается один модуль и его вставляем просто, без уменьшения. В ряду 19 модулей.


25 ряд — только желтые модули. В том месте, где вставляли 1 модуль (смотрите 24 ряд) нужно уменьшить одним модулем сразу 2 модуля. В ряду должно быть 18 модулей.
26 ряд — каждый третий модуль коричневый.
27 ряд — его нет на фото. Вставляем по два коричневых модуля. Мы начинаем делать коричневые зигзаги. В ряду 18 модулей.


28 ряд — Смотрите внимательно фотографии.
29 ряд — завершаем делать первый зигзаг.
30 ряд — его нет на фото. Между желтыми модулями вставляем коричневые модули, начинаем формировать второй зигзаг.


31 ряд — вставляем по два коричневых модуля.
32 ряд — между коричневыми вставляем желтый модуль.


33 ряд — вставляем по два желтых модуля и завершаем делать второй зигзаг.
34 ряд — только желтые модули.


35 ряд — увеличиваем количество модулей в два раза. В ряду 36 модулей.
36 ряд — вставляем модули через один.


37 ряд — вставляем только коричневые модули.


На этом заканчиваем делать вазу. Рекомендую ее проклеить клеем ПВА.
Как я уже писал ранее, Вы можете применить другие цвета при сборке вазы оригами.
Пишите Ваши вопросы в комментариях — отвечу с огромным удовольствием!
Здравствуйте уважаемые мастера и мастерицы, с вами Сергей Тарасов, и я представляю вашему вниманию новый мастер-класс по изготовлению вазы из модулей оригами в красно-черных оттенках. Я решил сделать плавный переход от черного к жёлтому и опять к черному. Как вы уже заметили, то на вазе оригами видны небольшие треугольные узоры, которые плавно перетекают от одного цвета в другой. Такое сочетание цветов придает особую выразительность вазе, а плавные переходы от одного цвета в другой – особую красоту и неповторимость.
Что самое интересное, то вы можете выполнить такую вазу в совершенно других цветах – в зеленых или синих оттенках. Главное, чтобы был плавный переход от одного цвета к другому.
И так, какие цвета нам понадобятся и в каком количестве. Чтобы изготовить такую вазу нужно приготовить 980 модулей, из них: черные — 216, темно-сиреневые — 244, красные — 188, оранжевые — 144, светло-оранжевые — 124, желтые – 64 модуля.


Высота вазы получилась 26 сантиметров и поэтому рекомендую делать ее модулей размером 1/16, если делать из модулей 1/32, то ваза получится довольно маленькая.
В процессе изготовления вазы оригами много раз приходится уменьшать количество модулей в ряду, и при этом я старался сохранить правильный рисунок. У основания вазы 48 модулей, а у самого узкого места, в горловине – всего 12 модулей. Следите внимательно за моими действиями, и вас все обязательно получится.
Модульное оригами ваза схема сборки.


Поведем сразу три ряда по 48 модулей. Первый ряд черные модули, второй ряд сиреневые и третий ряд черные.


В 4 ряду уменьшаем количество модулей с 48 до 32 модулей. Как это сделать? Нужно одним модулем захватить сразу три уголка и все это проделать по всему периметру без пробелов. Модули вставляем так, сначала сиреневый, потом 3 черных, один сиреневый и три черных – по всему кругу.


5 ряд. Сейчас мы начнем делать черные и сиреневые треугольники, в ряду 32 модуля.


6 ряд – продолжаем делать треугольники. Черные треугольники сужаются, а сиреневые расширяются. В ряду 32 модуля.


7 ряд – весь ряд из сиреневых модулей, в ряду 32 модуля.


8 ряд — красные модули. 9 ряд – только сиреневые модули.


10 ряд – начинаем делать красные и сиреневые треугольники. Смотрите, чтобы сиреневые треугольники были как раз напротив друг друга. В ряду 32 модуля.
11 ряд – продолжаем делать рисунок, в ряду 32 модуля.


12 ряд – уменьшаем количество модулей в ряду на 4. Уменьшаем там, где красные модули, только через один, то есть на одной красной вставке уменьшаем, следующую пропускаем и опять уменьшаем. В ряду должно быть 28 модулей.
13 ряд – только красные модули.


14 ряд – уменьшаем количество модулей на 4. Уменьшаем в том месте, где мы пропускали. Вставляем 2 оранжевых модуля с уменьшением, потом вставляем 4 модуля без уменьшения и так по всему периметру. В ряду должно быть 24 модуля.
15 ряд – только красные модули.


16 ряд – треугольники делайте симметрично, смотрите за моими действиями и повторяйте.
17 ряд – продолжаем делать красные и оранжевые треугольники.


18 ряд – только оранжевые модули, в ряду 24 модуля.
19 ряд – уменьшаем количество модулей на 4. Вставляем два светло-оранжевых модули с уменьшением, потом 3 модуля без уменьшения, далее 2 с уменьшением и так по всему кругу. В ряду 20 модулей.


20 ряд – только оранжевые модули.
21 ряд – следите за тем, чтобы треугольники были симметричны друг другу. Вставляем один светло-оранжевый и 4 оранжевых и так по кругу. В ряду 20 модулей.


22 ряд – уменьшаем количество модулей на 4. Вставляем светло-оранжевые модули и между ними уменьшаем оранжевыми модулями. В ряду 16 модулей.
23 ряд – продолжаем делать узор. Смотрите внимательно за моими действиями. В ряду 16 модулей.


24 ряд – только светло-оранжевые модули.
25 ряд – только желтые модули.


26 ряд – светло-оранжевые модули.
27 ряд – уменьшаем количество модулей на 4. Вставляем два светло-оранжевых модуля с уменьшением и один желтый. Повторяем по кругу. В ряду 12 модулей.


28 ряд – продолжаем делать узор, в ряду 12 модулей.
29 ряд – только желтые модули.


30 ряд – светло-оранжевые модули.
31 ряд – только желтые модули, в ряду 12 модулей.


32 ряд – из желтых модулей.
33 ряд – светло-оранжевыми модулями увеличиваем количество модулей в два раза. В ряду должно быть 24 модуля.


34 ряд – только оранжевые модули, в ряду 24 модуля.
35 ряд – красными модулями увеличиваем количество модулей в ряду до 36. Увеличиваем через один модуль.


36 ряд – только сиреневые модули, в ряду 36 модулей.


37 ряд – только черные модули.


Посмотрите на то, что у нас получилось. На этом этапе я бы посоветовал вам проклеить вазу, в таком виде горловина вазы может оторваться, лучше не рисковать. Еще вы можете из любого картона вырезать дно и приклеить.
На этом наш мастер-класс заканчивается, по всем вопросам пишите в комментариях.
Желаю удачи и до новых встреч.
Ваза из модулей оригами, Модульное оригами ваза схема сборки.
Модульное оригами ваза схема сборки
Схема сборки этой вазы очень проста, а может и сложна, в любом случае решать Вам. Схема сборки вазы имеет основательное, однородное дно, которое делается с самого начала сборки вазы.
И еще, эту вазу я сделал из необычных модулей, из модулей глянцевых журналов.


Нам потребуются модули размером 1/16. Как сделать модули из глянцевых журналов Вы можете посмотреть в видеоуроке «Как выбрать бумагу для модулей».
Еще нам понадобится клей ПВА и немного терпения, оно не помешает.
Перейдем к сборке вазы…
При сборке вазы смотрите внимательно фотографии. Модули из глянцевых журналов не имеют однородного цвета (пестрые) и при фотографировании они становятся менее заметными. Происходит слияние цветов, поэтому нет четкого изображения модулей.


1-2 ряд – делаем из 6 модулей в ряду.
3 ряд – увеличиваем в 2 раза – 12 модулей.


4 ряд – увеличиваем через 1 модуль. В ряду 18 модулей. Смотрите внимательно фотографии.
5 ряд – делаем так, 2 раза увеличиваем, потом просто вставляем модуль, потом опять 2 раза увеличиваем. В ряду 30 модулей.
Чтобы лучше понять и прочитать мои схемы рекомендую посмотреть этот видеоурок.
Продолжаем сборку вазы.


6 ряд – модули вставляем без изменений. В ряду 30 модулей.


7 ряд – увеличиваем в два раза каждый 3 модуль.
В ряду 40 модулей.


8 ряд – модули вставляем без изменений.
В ряду 40 модулей.


9 ряд – увеличиваем в два раза каждый 3 модуль.
В ряду 54 модуля.


10 ряд – без изменений. 54 модуля в ряду.


Делаем до 22 ряда – вставляем модули без изменений и придаем работе форму шара. В ряду 54 модуля.


23 ряд – уменьшаем количество модулей в ряду без пробелов, по всему периметру. В ряду 36 модулей.


24 ряд – модули вставляем без изменений. 36 модуля в ряду.


25 ряд – уменьшаем количество модулей через каждые 2 модуля.
В ряду 28 модулей.


26 ряд – модули вставляем без изменений. В ряду 28 модулей.


27 ряд – уменьшаем количество модулей без пробелов, по всему периметру. В ряду 19 модулей.


28 ряд – модули вставляем без изменений.


29-30 ряд – модули вставляем без изменений. Кончики модулей немного поднимаем вверх.


Делаем горловину до 34 ряда включительно. В ряду 19 модулей.
35 ряд – увеличиваем количество модулей в 2 раза. В ряду 38 модулей.
36 ряд – вставляем модули без изменений. В ряду 38 модулей.
На этом этапе желательно проклеить весь кувшин. Как лучше проклеить работу Вы можете посмотреть в этой статье.


Делаем подставку для кувшина из 40 модулей.
Наносим клей ПВА на подставку.


Сверху на подставку ставим сам кувшин. Смотрите чтобы кувшин стоял прямо.


На этом заканчиваем делать нашу вазу. Ждем пока высохнет клей полностью.
Не обязательно делать вазу из модулей глянцевых журналов. Вы можете сделать по этой схеме вазу из любых модулей и любого цвета.
По любому вопросу или пожеланию пишите в комментариях, отвечу с большим удовольствием!
Схемы [вазы]
Автор: Сергей Тарасов
30.10.2013


Предлагаю Вам еще один вариант изготовления вазы оригами из бумаги и не простой бумаги, а из глянцевых журналов. Очень много было прений по поводу этой вазы, дело в том, что это не простая ваза, а ваза-перевертыш, т.е. ее можно переворачивать…
Модульное оригами ваза

Если Вы хотите удивить близкого человека, друга или же просто хотите создать красивое оригами из модулей, мы предлагаем Вам схему сборки оригами «Ваза из модулей».
Эта поделка не очень сложная в сборке. Так что сам процесс принесёт Вам удовольствие, а сама поделка будет радовать глаз окружающих. В такую вазу Вы можете поставить цветы, собранные в технике оригами.
И так для поделки «Ваза из модулей» нам, как обычно, понадобятся, заранее приготовленные, модули. Цвет вазы, будет зависеть от цвета модулей. На данном примере, мы покажем сборку вазы из трех цветов, но учтите, что очень красивая ваза получиться даже если Вы замените цветную бумагу на обычную, белую, офисную бумагу, формата А4.
Для поделки Вам понадобятся 208 модуля: 152- белые; 92- зелёные; 64- жёлтые.
Начнём сборку!
Шаг 1. Начнём сборку с первого и второго ряда. Для этих рядов используем по 20 модулей белого и жёлтого цветов. Внимательно смотрим на картинку и собираем их таким же образом.
Шаг 2. Теперь приступаем к сборке третьего ряда.
Шаг 3. При сборке, мы, через каждый четвёртый модуль, надеваем один модуль белого цвета, только другой стороной.
Шаг 4. И так, у нас уже готовы три ряда поделки. Мы их аккуратно замыкаем в круг. Должна получиться такая поделка как на фото.
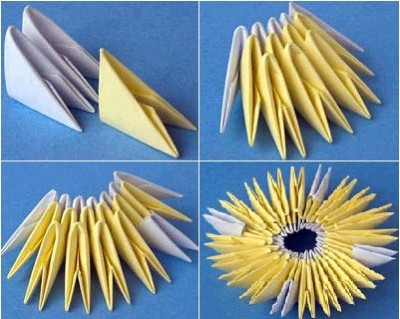
Шаг 5. Выворачиваем фигуру и добавляем еще один ряд, но только при этом увеличиваем число модулей белого цвета. Повторяем сборку так же как на рисунке.
Шаг 6. Далее добавляем следующий ряд.
Шаг 7. Затем, собираем еще два ряда.
Шаг 8. Добавляем еще два ряда и придаём вазе правильную, соответственную форму.

Шаг 9. Далее, берем модули белого и зеленого цветов и надеваем друг на друга, так как на фото.
Шаг 10. Сделанные заготовки, надеваем на модульную вазу.
Шаг 11. Посмотрите внимательно на рисунок и соедините таким же образом.
Шаг 12. На оставшихся участках повторяем так же.
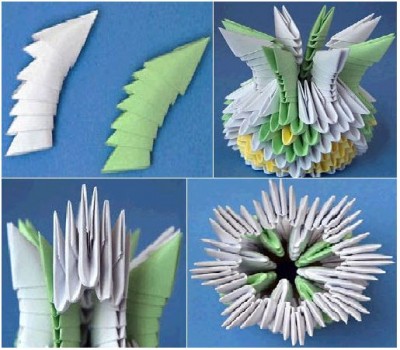
Шаг 13. Завершающим шагом будет сборка окантовки из зеленых модулей. Добавляем 32 зеленых модуля: надеваются они то на один, то на два угла.
Вот и все! «Модульная ваза оригами» готова!

Мы уверены, если Вы делали все так как указана в пошаговой инструкции, у Вас получилась замечательная и красивая поделка оригами. Ваза почти как настоящая. Жаль только воды нельзя налить 🙂
Еще, как дополнение к пошаговой инструкции, посмотрите детальный видео-урок по сборке «Модульной вазы оригами».
Спасибо Вам за внимание!
90000 Machine of modules. Origami: assembly diagram 90001 90002 Modular origami is becoming more popular inpresent time. Even children are able to create extraordinary masterpieces from these tiny details. Only because of its simplicity, which consists in copying small blanks and creative freedom, to spoil the product from paper particles is almost impossible. 90003 90004 Both old and small 90005 90002 This expression is ideal for the creativeThe process of creating various designs of paper modules.The fact is that he is subject to all who are able to sit on the ground for about an hour. But it is so much needed to create simple models of animals, people, heroes from fairy tales and cartoons, which somehow you can interest restless children. 90003 90002 If absolutely none of the abovelist did not arouse the interest of active youth, then we suggest assembling such a structure as a machine from modules. Origami, the scheme of which will be discussed in detail below. Its feature is that it is assembled on a plane, which allows to use it in the future as a picture for interior decoration.90003 90004 I blinded him out of what was 90005 90002 Before you start the process of creating a machine you need to carefully prepare the materials that you may need for further work: 90003 90014 90015 Paper. Perhaps, this is the most important thing that is useful in work. From its quality everything depends, from the flexibility of modules, to strength and durability. So, if a certain type of paper is very thin, then the probability that the product will crumble or break will increase several times.Especially if the children will take part in the process. The colors of the paper also play a big role, as the attractiveness and colorfulness of the crafts depends on them. 90016 90015 Glue. It is best to choose less toxic options, given that they will be contacted by children. Yes, maybe they do not glue so firmly and quickly, but it’s just a machine from origami modules, the scheme of which will be considered further, and not the world olympiad on bobsleigh. 90016 90019 90004 Minor materials 90005 90002 Also for work you will need: 90003 90014 90015 A more dense version of the paper is cardboard.If it is more convenient for you, then you can take a Whatman, which can later be cut into smaller sheets. 90016 90015 Scissors or clerical knife. Nevertheless, it is better to give preference to the first option, since it is less dangerous. In addition, there is a special type of scissors, designed only for children who are just starting to learn how to use them. Blades for them, often, are made of plastic, which makes them much safer. 90016 90015 Ruler. If this kind of measuring instruments was not found, then you can use a protractor or a triangle.90016 90015 If you are sufficiently versed in this direction of needlework, then it’s easier for you to rely on modular origami assembly schemes, rather than step-by-step instructions that take longer. 90016 90019 90004 Modular origami machine: assembly diagram 90005 90002 The assembly scheme of the product is very simple and understandable, especially if you look at it step by step. So, this process will be performed by the child himself. 90003 90002 Prepare paper fragments for furthercreating a shape.Since this is a machine from modules, origami, the scheme of which is conspicuous, bright and juicy shades should be used. So, for the wheels and strokes you will need black parts, for glasses and metal parts — white or blue versions, and the base of the machine you choose, based on personal taste. In our case, red. 90003 90040 90015 Begin the process of assembly from the bottom of the picture. To do this, we carefully consider the circuit, consider how many modules of different colors we need, and put them close to each other on a flat surface.Usually the first row is not fixed. But if it is more convenient for you, then grind the parts among themselves and carefully lay them out in the right order. 90016 90015 It’s time to proceed to the next row. Modular schemes of assembling origami are specially created in such a way that the buyer, whether he is an avid creator of paintings from paper or just interested in a beginner, was simple and understandable while working with the picture. Therefore, you can easily find out what you need for the second row and fix the details in the right places.Again, if you prefer, then fix it with glue, and then dry the rest of the fragments in the desired position, so that there are no excess bends and creases. 90016 90015 So, row by row recreate the machine according to the scheme. The number of modules should not change throughout the process, and if this happens, then it is necessary to count all previous series and, having found the error, disassemble to the correct number and fix everything. 90016 90015 When all the ranks are finished, then you can carefullyglue the back side to give the product strength and strength.As it turned out, origami «Machine» of modules, the scheme and the whole process of creating which is extremely simple, you can add yourself. 90016 90049 90004 To decorate 90005 90002 We did not in vain learn to make modular origami «Machine», the scheme of which turned out to be not so complicated as one might think. Now this hand-made thing can decorate the room, turning it into a picture, a stand under the organizer. Also, you can just give it to family and friends. 90003 .90000 Modular origami. Interesting schemes of assembling decorative plates 90001 90002 90003 Modular origami, assembly scheme of decorativeplates — an excellent option for a gift to loved ones. Modular origami is assembled on the basis of basic triangular modules. Assembly schemes allow you to learn how to collect the proposed variant of decorative plates and in the future to modify the model, experiment with shapes and colors.Tips for assembling decorative plates An amazing way to create a unique decor for your home is modular origami. Schemes of assembly of decorative plates are presented in order for beginners to master the skills to collect three-dimensional figures. The use of a special assembly technique will create such a decorative plate. 90004 A few mysterious transformations and intricate product will become more attractive, original, will acquire an unusual decor, as in this photo. 90005 For an embodiment of a decorative plate, which was listed above in the first photo, you will need about 1000 modules.It is not necessary to immediately prepare the full number of blanks. They can be twisted from a suitable sheet of paper during the assembly of the product. If there is a desire, you can change the color of the module, and when you acquire good assembly skills, you create the plates of a unique shape. In total, white, blue and yellow modules will be needed for the first version of the dish, in the number of 356, 301 and 339, respectively. To produce a more complicated version of the diamond-decorated dish, 636 white modules, more than 500 blue and yellow shades, will be needed.At the first stage, it is necessary to assemble a chain of 42 pieces of the same color and to close it in a circle. Thus, it is necessary to make two rows. In the next row, the assembly is performed with alternating colors — 1 yellow element, five blue modules. Then add the yellow modules and reduce the amount of blue, that is, alternate 2 elements of yellow and four blue. When assembling the next series, it is necessary to reduce the number of blue modules again, and collect the yellow elements only at the edges, so that the middle remains unfilled.When angular fragments are formed during assembly, they must be connected by a white module. At this stage of the assembly, the figures should appear, as indicated in the diagram. 90006 Now proceed to the white workpiece. They gradually spread out so as to rise in height to the top of the formed corner element of the plate. Get a figure from inside, like this image. 90007 Now between each corner fragment we form a column of six modules and build up the vertices using also six modules. Throughout the perimeter of the plate will turn out arches.See the diagram. 90008 White modules connect to an angular fragmentblanks of white color. Then a series of yellow and blue modules is assembled. So we approached the assembly of the first version of the plate. Experienced artists are attracted by modular origami, the assembly scheme of decorative plates which depicts colored rhombs along the edge. For this it is necessary not to stop, but start filling in the fragments between the modules, as in this scheme. 90009 In the last row of the blue modules we form sharp corners.90010 90011 Comments 90012 90003 comments 90010 .90000 Origami Vase Folding Instructions 90001 This is a really nice traditional origami vase. The end result looks much more complicated than it actually is. 90002 90003 90002 Made this origami? Comment and Submit your photo using the comment box at the end of this page! 90003 90002 90003 90002 90003 90002 Origami Vase Step 1: You can start with a 6 inch x 6 inch (15cm x15cm) square origami paper, color side down.90003 90002 In order to make a larger vase, we are actually using a 21 cm x 21 cm square origami paper. 90003 90002 Divide you paper into thirds both horizontally and vertically. Fold, crease well and unfold. 90003 90002 90017 90003 90002 90020 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 2: Now divide your thirds in half, both horizontally and vertically. Fold, crease well and unfold. 90003 90002 90031 90003 90002 90034 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 3: Divide your creases in half again, both horizontally and vertically.Fold, crease well and unfold. 90003 90002 You should end up with 12 small «squares» on each row and column. 90003 90002 90047 90003 90002 90050 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 4: Fold paper from the right to left. The crease is at 5 squares from the right. 90003 90002 90061 90003 90002 90064 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 5: Fold paper back to the right.The crease is at 1 square from the right. 90003 90002 90075 90003 90002 90078 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 6: Now fold paper from left to right. The crease is at 5 squares from the left. 90003 90002 90089 90003 90002 90092 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 7: Fold paper back from the right to the left.The crease is at 1 square from the left. 90003 90002 90103 90003 90002 90106 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 8: Now fold paper from the bottom to the top. The crease is at 5 squares from the bottom. 90003 90002 90117 90003 90002 90120 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 9: Fold paper back from top to the bottom. 90003 90002 90131 90003 90002 90134 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 10: Fold paper from the top to the bottom this time.90003 90002 90145 90003 90002 90003 90002 90150 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 11: Finally, fold it back from the bottom to the top. 90003 90002 90161 90003 90002 90164 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 12: Flip paper over. 90003 90002 90175 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 13: Starting from the top, we’ll make 2 diagonal mountain folds.Crease well and unfold. 90003 90002 90186 90003 90002 90003 90002 Closer view: 90003 90002 90193 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 14: Pry paper open and make a squash fold on each side by pressing paper flat. 90003 90002 90204 90003 90002 90207 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 15: Repeat steps 13 and 14 for the other 3 sides.90003 90002 90218 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 16: Flip paper over. 90003 90002 90229 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 17: Make diagonal folds on each of the 4 sides. Crease well and unfold. 90003 90002 90240 90003 90002 90243 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 18: Fold right and left sides to the center.90003 90002 90254 90003 90002 90257 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 19: Fold the bottom half to the center. Tuck the paper into the «pocket». 90003 90002 90268 90003 90002 90271 90003 90002 90003 90002 closer view of tucking paper into pocket …. 90003 90002 90278 90003 90002 90281 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 20: Now fold the top half to the center.Once again, tuck paper into the pocket. 90003 90002 90292 90003 90002 90295 90003 90002 90298 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 21: Almost done! Make diagonal mountain folds to create an opening for your vase. 90003 90002 90309 90003 90002 90312 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 22: Flip paper over. 90003 90002 90323 90003 90002 90003 90002 90003 90002 90003 90002 Origami Vase Step 23: Now the transformation begins! Start to gently pry paper out of its fold on all sides.90003 90002 90334 90003 90002 90337 90003 90002 90003 90002 As you pull the paper out, suddenly your «flat» paper becomes a 3D object! This is what we love about origami !! 90003 90002 90344 90003 90002 90003 90002 Flip paper over and your origami vase is complete! 90003 90002 90351 90003 90002 90354 90003 90002 90357 90003 90002 90003 90002 Fill it up with origami flowers! 90003 90002 90364 90003 90002 90367 90003 90002 90003 90002 90003 90002 Did you make this origami? If so, 90374 upload your photo 90374 (2MB limit) 90376 via the comment box below 90376.You can login with your Facebook, Twitter, Google or Yahoo accounts. 90003 90002 90003 .90000 Peacock from origami modules: assembly diagram 90001 90002 If you have a significant amount of patience andassiduity, then any applied creativity, including origami, is up to you. Creating crafts from paper — creativity, whose age is hundreds of years. Origin of origami is Japan, which many centuries ago, Chinese monks brought the paper. And only in the middle of the nineteenth century the art of folding paper figurines came to Europe. 90003 90004 Modular origami 90005 90002 One of the most popular areas of origamiis considered modular.The first mention of modular origami dates back to 1710. Learn this skill is not as problematic as it seems at first glance. The main thing here is to understand the technique of adding one module. And then to make of an individual paper units an unusual flower, an elegant bird or an animal will not be so difficult. 90003 90002 If in ordinary origami the figure consists of only one leaf, then in the modular «takes part» a multitude of multicolored or monophonic sheets, folded in a special way. 90003 90002 We suggest that you stock yourself with a bit of patience andmaster this technique.Theme today is «Peacock from the modules». The master class will consist of learning the technique of folding a single module and a scheme that will help to collect a beautiful bright bird. We will offer several photovariants, which color scale and technique to adhere to — everyone will already decide for themselves. 90003 90004 Make the module 90005 90002 To begin, let’s learn how to make singledetails — modules. Any figure in origami consists precisely of them, and the peacock of the modules will also be added.A step-by-step instruction is presented in the photo, but we will add a few explanations in words. 90003 90002 To make a beautiful figure in technologymodular origami, you need a piece of paper. Aspect ratio must necessarily be one to two. That is, one side should be exactly twice as long as the other. Depending on the size of the sheet, the size of the module and the craft itself will change. 90003 90002 The first thing to do is to fold the leafletin half. Then, outlining the center line, fold the corners of the paper to the center.We turn our workpiece and lower edges also bend to the bottom of the module. Now bend the bottom part up completely. The last step is to fold the triangle in half and it’s ready. Now in the formed «pockets» we will insert one triangle into another. And thus we will have a beautiful peacock from origami modules, the scheme of which is understandable and simple. 90003 90002 How many triangles withpockets? How many blanks are needed? Peacock from origami modules, the scheme of which will be presented a little later, can have up to a thousand or more such blanks in its composition.It will remain to determine the color gamut and to procure such a number of modules of a certain color, which is necessary for each row of origami. 90003 90002 Tip: when you decide on the color scheme, then make a little more blanks of each color. Sometimes in the process of working the modules can be torn or deformed, and then they are no longer usable. The stock must always be there. We make more triangles so that we have a nice peacock from the origami modules (without any problems and time costs).90003 90004 What will you need? 90005 90002 We will offer you our color scheme, but shecan be absolutely any. If you are planning that a peacock of origami triangular modules «settle» in your living room or a nursery, then the color scheme can be chosen so that the article is worthy of elements, successfully inscribed in the interior of the room. If you make it as a gift, then you can choose any color solutions that are just «to your liking» and «to your liking». 90003 90028 90029 856 yellow blanks; 90030 90029 465 — green; 90030 90029 114 white modules; 90030 90029 one red triangle for a spout; 90030 90029 one black triangle; 90030 90029 glue.90030 90041 90002 We will make a reservation at once. We offered the simplest set of colors to make it convenient to talk about how to make a peacock out of modules. But you try to make the color scheme even richer, especially in the place where the tail is located. Then the bird will turn out really graceful and bright. 90003 90004 First circles 90005 90002 Determine with the size and make the first circle, inserting one triangle into the pocket of the neighbor. Thus, in yellow, we will form three circles.Our initial circle will consist of the 31st triangle. In the fourth round, we will add other shades of «feathers». We do the following: the first green, the next yellow, then white, five yellow, one white, one green, the remaining yellow. 90003 90004 Doing down 90005 90002 Let’s say right away that everyone has different views, how the peacock should look from the origami modules. The scheme can be borrowed from the Internet or drawn by you personally. Therefore, reduce in the area of the tummy do as shown in your diagram.We will do this after the fourth row and up to the eighth. 90003 90002 To do this, we turn the model over and insert the triangles so that in the subsequent rows, one blank becomes smaller. Do not forget to observe the order of the colors given in the fourth row. The body is ready. 90003 90004 Breast 90005 90002 Now it’s the breast turn. Peacock from origami modules, the scheme of which is proposed by us, has the same coloring in the chest area, as in the abdominal region. Therefore, we make the first row like this: the first triangle is green, three yellow, one green, three yellow and one final green module.Now you will need to make eight more rows, making a reduction in the neck area. 90003 90004 Neck 90005 90002 The neck is one of the most elegant parts, of whichconsists of any peacock from origami modules. Scheme and design of the neck in all models is similar to each other. At us it will consist of seventeen rows. We divide them in this way: even rows and odd. All the even ones will consist only of white triangles, there will be two. But the odd numbers are made up of three triangles: yellow — the first, then — green, the final — yellow.The last row is only white modules. 90003 90004 Head 90005 90002 The first three rows are done exactly in the same order, as they did on the neck. The fourth consists of two green blanks, the fifth — from one green triangle. Now it will be necessary to fix the spout, that is, add black and white modules at the end of the neck. 90003 90004 Tail and wings 90005 90002 As for the tail and wings, here we wouldadvised you to make a combined model. Take a few options and connect them together. The tail starts with eight or nine modules and then increases.Try to add workpieces gradually, so that the design is symmetrical. 90003 90002 As for the wings, there will be moreeasier. They begin, as a rule, with one of two triangles, then the attachment goes to two or three in one row. The upper, most fluffy part of the wing, can include up to fifteen modules. So our peacock is ready from the modules. The assembly scheme is simple, hopefully, it is described clearly. Everyone will cope with the task, even a beginner, who decided to train his patience. 90003 90004 Stand 90005 90002 It remains to make an elegant stand, on whichwill be located peacock of the modules.The scheme for creating such a support resembles the first rows of the peacock’s body. That is, we make a circle of thirty modules, then add a few more rows. We insert the first rows of the body into the last rows of the support. 90003 p >> .